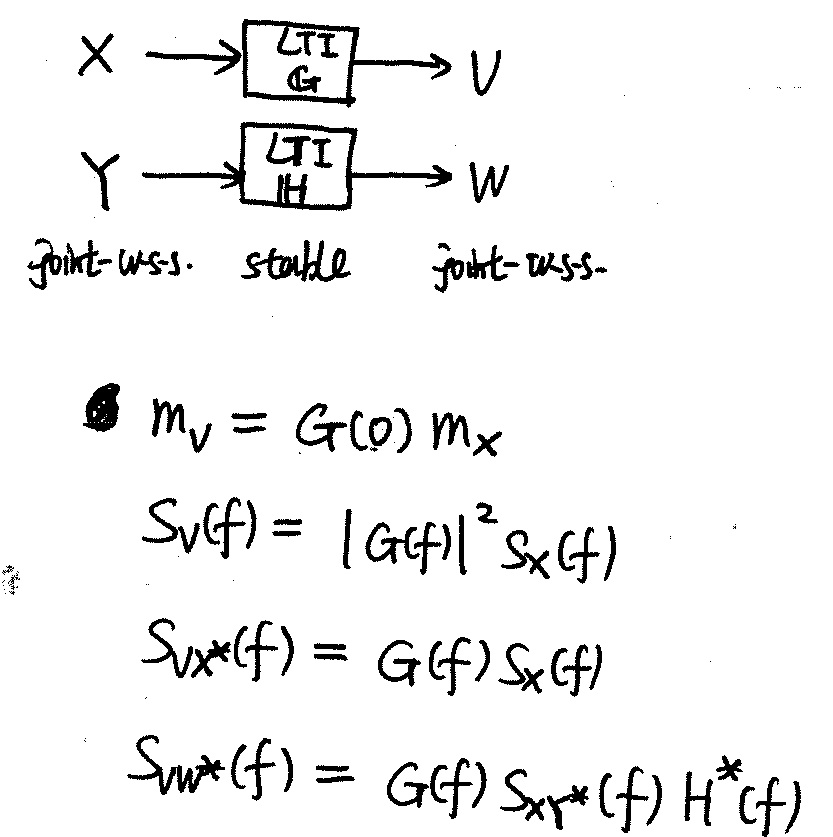System
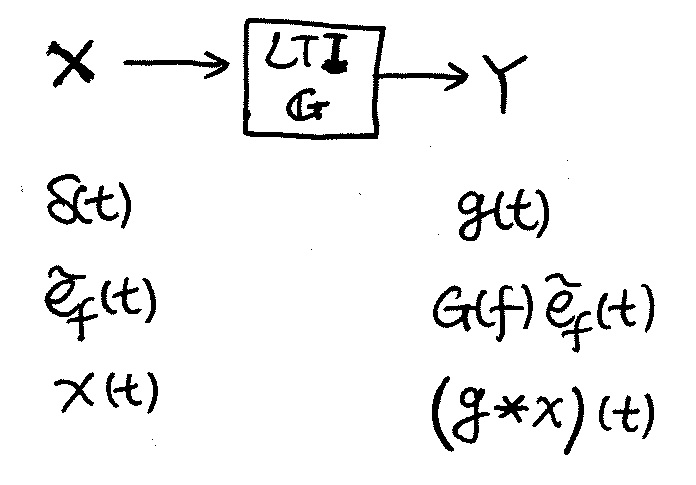
Linear Time/Translation Invariant (LTI) Operators (Chap. 22.1)
- e-function: \( \tilde{e}_f(t) = e^{i2\pi ft} \)
- \( G(f) = \mathcal{F}{ g(t) } \)
- stable: bounded input produces bounded output
- Given \( \mathbb{H} \) is LTI. Stable is equivalent to \( h(t) \) absolutely integrable.
- Given \( \mathbb{H} \) is LTI. Causal is equivalent to \( h(t)=0, \forall t<0 \).